Abstract
Water is a polar liquid and has a dielectric permittivity much higher than typical apolar liquids, such as light oils. This strong dielectric contrast at water-oil interfaces affects electrostatics and is important, for example, to include these effects to describe biomolecular processes and water-oil mixtures involving surfactants, as detergents. In this pilot project, developed in collaboration with Unilever and Manchester University, we have proposed and analysed a class of polarisable solvent models to be used in Dissipative Particle Dynamics (DPD), a coarse-grained particle-based simulation method commonly used in various industrial sectors. Related software modules for the DL_MESO package have also been developed.
Silvia, could you tell us a bit about yourself, where do you come from originally and what brought you to simulation and to work with E-CAM?
I am originally from Italy, and my background is in Theoretical Physics. I am mostly interested in Statistical Physics, and my research and teaching activities brought me towards simulations and in contact with E-CAM.
What are the goals of your pilot project? Why is this project important in general scientifically, and from an industry perspective in particular, and how is E-CAM helping Unilever to solve this problem?
Our aim is to design a realistic mesoscale model for water, computationally efficient and well described by liquid-state theory. A key feature of water that such a model must capture is, among others, polarisability. Dissipative Particle Dynamics (DPD) [1] is a method to describe fluids at a mesoscale level: in this approach, both the solvent and the solutes are modeled on a similar footing (i.e., as particles) and hydrodynamic behaviour is correctly reproduced. The modelling of polarisable solvents at this scale is important whenever electrostatic interactions play a relevant role in the system and all-atomistic calculations are computationally too expensive or not feasible. To corroborate our results, we compare different numerical approaches [DPD and Monte Carlo (MC) simulations] and liquid-state theory predictions.
Oil-water mixtures (dispersions, emulsions, also involving solutes and surfactants) are present in a variety of industrially relevant problems, for example in oil extraction, pharmaceutics, food, house-hold and personal care products. E-CAM is participating in the development of the models and their testing, and coding related software tools.
Can you elaborate a little bit more on your approach and explain to what extent your software is built on what was there before and to what extent there are new things?
It is necessary to keep a balance between computational efficiency (e.g., few charges) and an accurate description, ensuring at the same time the model predictivity.
Traditionally, particle-based simulations use permanent fixed point-like charges. However, flexible charge distributions are needed in many cases, and polarisability has been recently addressed in atomistic and coarse-grained models using various methods. [2]
In a DPD simulation, there are two main approaches to include a dielectric medium (say, water): one can either impose a certain permittivity (implicit static medium), or include dynamic microscopic objects which carry a charge dipole moment and give rise to that permittivity (explicit medium). Using both, we can split the medium effect in a background (implicit) and an explicit term: this is our choice, and the typical system we have into mind is water in background oil [3]. A third (implicit dynamic medium) approach is also possible [4], but a bespoke method is needed for electrostatics and there is the drawback that the forces on neutral particles are neglected in the original formulation.
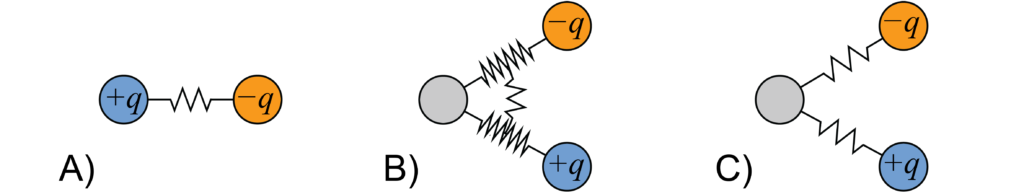
Polarisable water models for DPD have been recently proposed by Peter and Pivkin [5] and Vaiwala et al. [6]. In our project we have analysed three classes of explicit solvent models: harmonically-bonded dimers (+q,−q), and different trimers (+q,0,−q) [Peter-Pivkin‑like models, and double-spring (DS) trimers, where the springs are soft and the harmonic angular bond is removed, see the pictorial representation in Figure 1].
Figure 1: Sketches (not in scale) of the models considered for the polarisable solvent. A) Dimer, B) Peter-Pivkin–like trimer, and C) double-spring trimer. [Image credits: Dr. M. Mališ]
As compared to the models available in the literature, we propose a correction of Peter-Pivkin parametrization, and one of our models is similar to that of Vaiwala, with the main difference that we do not switch off intra-molecular electrostatics. Our DPD mapping is such that three water molecules correspond to one bead for dimers, whereas they correspond to three beads for trimers. It is important to underline that our model, in a top-down approach, aims at matching the dielectric properties at the continuum scale, not the microscopic one. In other words, the solvent will be a medium of given permittivity at scales larger than the bead size, whereas effects below this scale are artefacts due to discretisation. Our models allow the use of existing DPD codes and methods for electrostatics (such as Ewald summation), and are supported by liquid-state theory predictions, which allows for a faster parameter adjustment. Once a parameter set has been identified thanks to the guidance provided by liquid-state theory approximations, the dielectric permittivity of the medium is computed in MC and DPD from dipole moment fluctuations, according to linear response theory. We have tested the dielectric behaviour of the solvent in various situations: the response to an external electric field, the polarization charge around an ion, the force between two test charges and the ion desorption from an oil-water interface. All the analysed models show a similar phenomenology as dielectrics, however the DS-trimer is probably to be preferred since it entails a minimal modification to the non-polar water fluid, and is well described by liquid-state theory.
Can you outline the software that you developed during your pilot project? Can people add functionalities to your codes and what would they need to do?
The base code for the software I have developed is the DPD code of DL_MESO, [7] a general purpose mesoscale simulation package developed by Dr. M. A. Seaton at Daresbury Laboratory (STFC, UK). The main software modules developed so far analyse charge dipole moments properties, such as the Kirkwood factor and the solvent permittivity (gen_dipole.f90), dipole-dipole autocorrelation functions (gen_dipoleaf.f90, gen_moldipaf.f90). Work is in progress on a module to analyse tetrahedral ordering (due to hydrogen bonding) and the response to spatially modulated electric fields.
Since the codes (all open source) are properly commented and individually documented according to E-CAM best programming practices, I think users can easily add their own functionalities to them.
Are your codes already being applied to the systems of interest? Are they being tested by the industrial partner?
Yes, the tools have been used by four Master of Engineering students, supervised by Prof. A. J. Masters at the University of Manchester, as part of their dissertation projects. The DPD results are, whenever possible, validated by comparison to those obtained with completely independent codes (for MC and liquid-state theory) by Dr. P. B. Warren, who is a staff scientist for our industrial partner, and is a member of this project.
What do you think are the future prospects and likely impact of this project?
We plan to apply these models to the self-assembly of ionic surfactants, as SDS (Sodium dodecyl sulfate), [8] which is an industrially relevant problem, to explore the effect of the dielectric contrast between the micelles core (oil) and their surroundings (water).
Are there any publications which describe the method in more detail?
Yes, we are finalizing a publication [9] describing the proposed models and showing their behaviour in a series of test cases.
